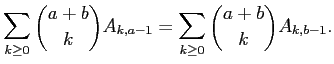Séminaire Lotharingien de Combinatoire, B67c (2012), 11 pp.

Guo-Niu Han, Zhicong Lin and Jiang Zeng

A Symmetrical q-Eulerian Identity

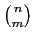Abstract. We find a q-analog of the following symmetrical identity involving binomial coefficients $ \binom{n}{m}$ and Eulerian numbers An,m, due to Chung, Graham and Knuth [J. Comb., 1 (2010), 29-38]:
$\displaystyle \sum_{k\geq 0}\binom{a+b}{k}A_{k,a-1}=\sum_{k\geq 0}\binom{ a+b}{k}A_{k,b-1}.$    
We give two proofs, using generating function and bijections, respectively.

Received: February 17, 2012. Accepted: March 17, 2012. Final Version: March 23, 2012.

The following versions are available: